Online Professional Development Outline - FENLS Grades 6-8
The following Online PD Outline can be reviewed by individual teachers, discussed as part of a PLC meeting, or used as the inspiration for a short math workshop.
Apply Measurement Strategies to Number Lines and Percent Problems
Overview
Students who lack basic number line strategies will struggle to solve many middle school math problems. This Online PD Outline will review one of the four essential number line strategies, Basic Measurement, so that teachers can recognize, reteach, and help students apply this strategy.
Professional Development Goals:
-
Recognize that some students do not understand the basic rules for how number lines work
-
Investigate ways to reteach number line strategies that emphasize mathematical reasoning
-
Apply number line strategies to solve a percent problem
-
Explore how number-line-based diagrams form the basis for more formal percent strategies
PLC Meeting Notes (pdf) - Make copies for teachers to take notes when using this PD Outline as part of a PLC Meeting
PD Agenda and Facilitator Notes - Run a professional development session based on this PD Outline (Coming Soon)
Note, this PD Outline focuses on the most basic number line strategy, measurement. Online PD Outlines for the Split Distance, Base-10, and Approximation Number Line Strategies will be available in Spring 2018.
Do My Students Know How Number Lines Work?
The following number line problem and video will help teachers to review some common mistakes that students make when working with number lines. Teachers can download a copy of this number line problem to try it with students in their class.
Assessing Students' Understanding of the Basic Measurement Strategy
The following problem can be used to quickly assess which of your students understand the basics of how number lines work. It also provides an opportunity to introduce and apply an essential number line concept, the Basic Measurement Strategy. Download a PDF with four instances of this problem here. The PDF can be printed, copied, and cut into four strips to save paper.
The problem below can be given to a set of students in grades 3 and above. DO NOT show students how to solve the problem or review number line strategies before giving them the problem to solve. This will allow you to assess which students understand the basics of number lines and which students do not.
Before moving on to the next section and watching the video, ask yourself the following question:
What incorrect solutions do you think students might come up with when solving this problem?
Incorrect Student Strategies
The video below walks through some of the incorrect solutions that students come up with to label the open tick marks on the number line above. Note, students that struggle with number sense are most likely to struggle with labeling the open tick marks in this problem. But, don’t be surprised if more advanced students come up with incorrect solutions or struggle to justify their correct answers.

Use Measurement Strategies to Justify Number Placement
The two videos below review how measurement strategies are used to locate points and tick marks on a number line and justify why the placement of a given value is correct or incorrect.
Use the Basic Measurement Strategy to Label Open Tick Marks
The number line problem above can be used to introduce the Basic Measurement Strategy:
The distance between two labeled points (or tick marks) on a number line can be measured
and used to locate other values on that number line.
The emphasis in this video is on providing students with a number line strategy or “rule” that they can apply to justify the location of values on a number line.
Use a Measurement Strategy to Show that the “Skip Count” Solution is Incorrect
Not only can the Basic Measurement Strategy be used to determine the correct value of points and tick marks, it can be used to show that a specific value is labeled incorrectly. Once again, the emphasis is on getting students to use mathematical reasoning and justification when working with number lines.
Apply Number Line Strategies to Solve Percent Problems
The following videos extend number line measurement strategies to solve common middle school percent problems.
In addition, the last two videos will demonstrate how number line based diagrams, such as percent bars, are an important building block for more formal percent concepts and strategies such as equivalent ratios, multipliers, and proportions.
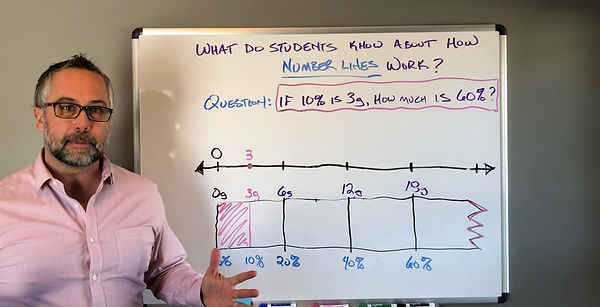
Apply a Measurement Strategy to Solve a Problem Using a Percent Bar
Students that are familiar with number line strategies can apply them to solve a host of middle school math problems, including problems involving percents, ratio, rates, algebra, geometry and statistical representations. In the video below, number line strategies are applied to solve a common percent problem using a percent bar.
Use a Number-Line-Based Diagram to Introduce Formal Percent Strategies
Number-line-based diagrams, such as percent bars, can be used to introduce more formal percent strategies such as equivalent ratios, multipliers, and proportions. This helps demonstrate why number lines strategies form a foundation for proportional reasoning and are essential to success in middle school mathematics.
This first video explores the equivalent ratios that are set up when building a percent bar and shows how the multiplicative relationship between these ratios can be used to solve a percent problem.
Note, this video demonstrates the use of one number line strategy on a percent bar to solve one type of percent problem. Other percent problems with different structures can be solved using other number line strategies and percent bars. These problems and strategies will be investigated in other MTF Online PD Outlines.
This second video demonstrates how the values on a percent bar can help students to correctly structure a proportion that can be used to solve a percent problem. In this case, the number-line-based diagram can help students understand how proportions should be structured and apply that understanding to solve percent problems.
Note, there is more than one way to structure a proportion using a percent bar. This video only covers the most basic and intuitive approach. The other structures are also important but are not covered in this Online PD Outline.
Feedback - Online PD Outline